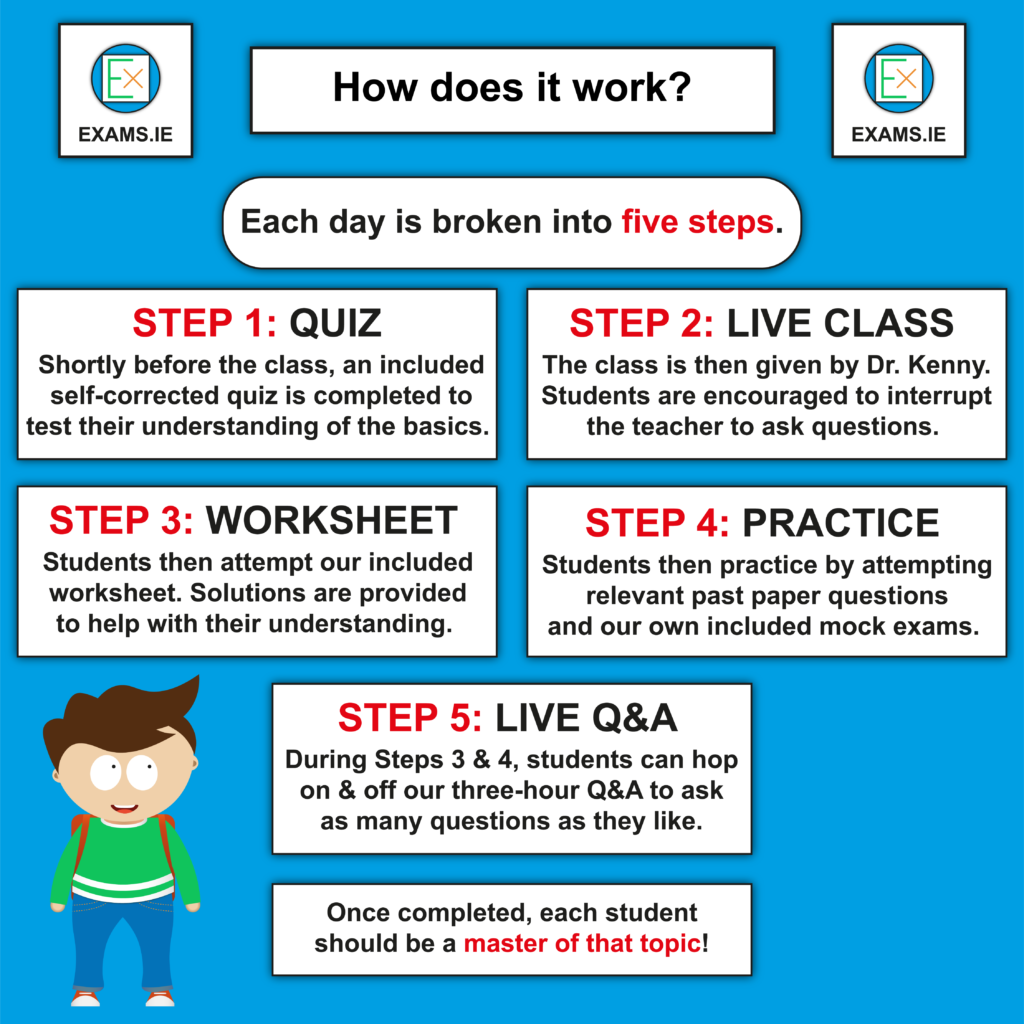
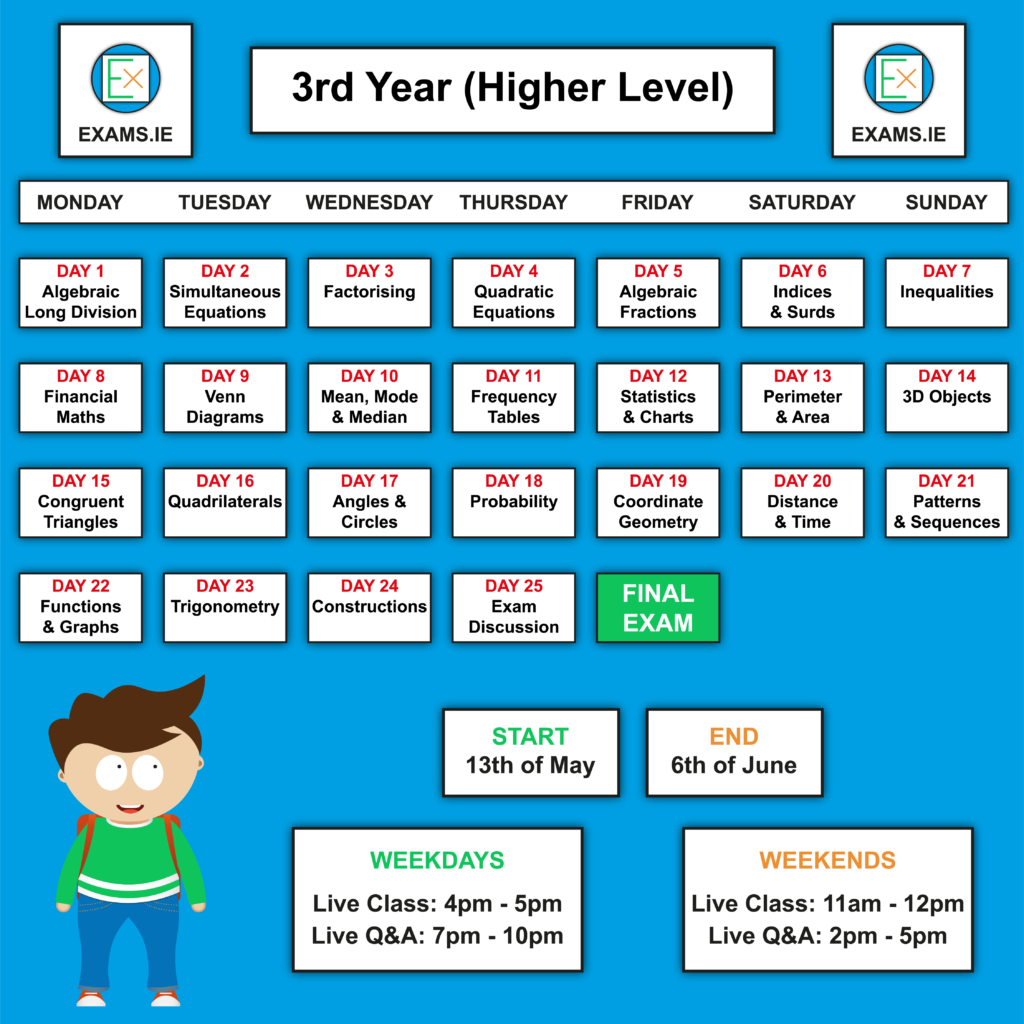
About Lesson
Natural Numbers
0/14
Integers
0/6
Fractions
0/8
Probability
0/4
Decimals
0/8
Sets
0/9
Percentages
0/8
Algebra
0/8
Length, Area & Volume
0/10
Geometry 1
0/4
Ratio & Proportion
0/9
Statistics
0/3
Coordinates
0/5
Solving Equations
0/8
Geometry 2
0/8
Visualising Data
0/6
Transformation Geometry
0/4